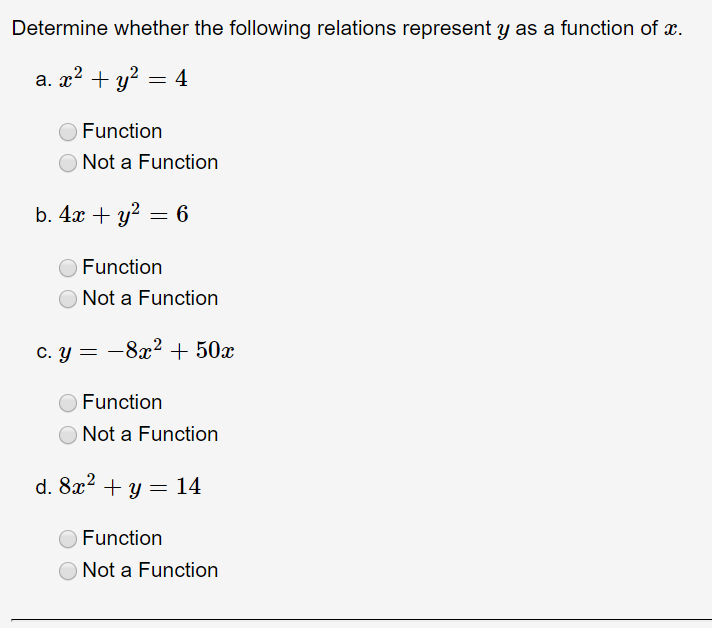
Is x2 y2 4 a function or not
This is a many-to-many relation because a single x-value relates to two different y-values. Therefore x2+y2=4 is not a function.
Is any equation a function
A function is an equation that has only one answer for y for every x. A function assigns exactly one output to each input of a specified type. It is common to name a function either f(x) or g(x) instead of y.
What is a function and what is not
This is a non-function. For mapping diagrams. I'm going to use my same definition every x can i have only one y. Value.
What type of function is y =- 2x
So x is zero y is zero. So we find x is zero. And then y is zero right there how about x is one y is two so we go one and then we go up two. And then x is negative one and y is negative 2.
How do you tell if a is a function
Determining whether a relation is a function on a graph is relatively easy by using the vertical line test. If a vertical line crosses the relation on the graph only once in all locations, the relation is a function. However, if a vertical line crosses the relation more than once, the relation is not a function.
How do you determine if this is a function
Use the vertical line test to determine whether or not a graph represents a function. If a vertical line is moved across the graph and, at any time, touches the graph at only one point, then the graph is a function. If the vertical line touches the graph at more than one point, then the graph is not a function.
What equation is not a function
Horizontal lines are functions that have a range that is a single value. Vertical lines are not functions. The equations y = ± x and x 2 + y 2 = 9 are examples of non-functions because there is at least one -value with two or more -values.
Is this a function or not
Use the vertical line test to determine whether or not a graph represents a function. If a vertical line is moved across the graph and, at any time, touches the graph at only one point, then the graph is a function. If the vertical line touches the graph at more than one point, then the graph is not a function.
How do I know if it’s a function
If I put another point right here for example. What. It means is that for this x-value. For I'm getting a y-value of 1.
Why is x2 y2 not a function
Since there exists two values of y for a single value of x therefore it is not a function as in a function, there must be only one existing value of y for a single value of x. Was this answer helpful
Why y2 is not a function
It is not, because the definition of a function includes the requirement that no x will have more than one corresponding y. The parabola you named has its vertex at (0,0) and opens to the right, so every x value > 0 will have two corresponding y values, one positive and one negative.
What is the easiest way to identify a function
So this one is a function. So we're gonna say yes it is a function. If you happen to draw a vertical line on your graph.
How do you tell if a function is a function or not on a graph
And different y-coordinates the vertical line goes through two points on the graph. And what that means is that this graph does not represent a function.
How do you know if it is a function
More. Than one point for a given vertical line now when you scan across here you can see that's never the case every time you know I draw a vertical line it's only crossing at most one point whereas.
How can I identify a function
To identify a function from a relation, check to see if any of the x values are repeated – if not, it is a function. If any x values are repeated, and the corresponding y values are different, then we have a relation and not a function.
How do I identify a function
To identify a function from a relation, check to see if any of the x values are repeated – if not, it is a function. If any x values are repeated, and the corresponding y values are different, then we have a relation and not a function.
How do you check if it is a function
Use the vertical line test to determine whether or not a graph represents a function. If a vertical line is moved across the graph and, at any time, touches the graph at only one point, then the graph is a function. If the vertical line touches the graph at more than one point, then the graph is not a function.
How do you know if it’s a function or not without a graph
How do you figure out if a relation is a function You could set up the relation as a table of ordered pairs. Then, test to see if each element in the domain is matched with exactly one element in the range. If so, you have a function!
How do you tell if an equation is not a function
A function will only have one or zero outputs for any input. If there is more than one output for a particular input, the equation does not define a function. In order to be a function, each value of should have, at most, one output value of .
How do you tell if it’s a function
Use the vertical line test to determine whether or not a graph represents a function. If a vertical line is moved across the graph and, at any time, touches the graph at only one point, then the graph is a function. If the vertical line touches the graph at more than one point, then the graph is not a function.
How do you identify if it is a function
How do you figure out if a relation is a function You could set up the relation as a table of ordered pairs. Then, test to see if each element in the domain is matched with exactly one element in the range. If so, you have a function!
How do you identify a function or not a function
If any vertical line drawn can cross the graph at a maximum of one point, then the graph is a function. If there is any place a vertical line can cross the graph at two or more points, the graph is not a function.
What graph is not a function
If you can draw a vertical line any where in the graph and it crosses more than 1 point on the graph, then the graph is not a function.
How to tell if a graph is a function yes no why or why not
Inspect the graph to see if any vertical line drawn would intersect the curve more than once. If there is any such line, the graph does not represent a function. If no vertical line can intersect the curve more than once, the graph does represent a function.
How do you prove a function is a function
Notice that to prove a function, f : A → B is one-to-one we must show the following: (∀x ∈ A)(∀y ∈ A)[(x = y) → (f(x) = f(y))]. This is equivalent to showing (∀x ∈ A)(∀y ∈ A)[(f(x) = f(y)) → (x = y)].