What is the percentage of 1 standard deviation
around 68%
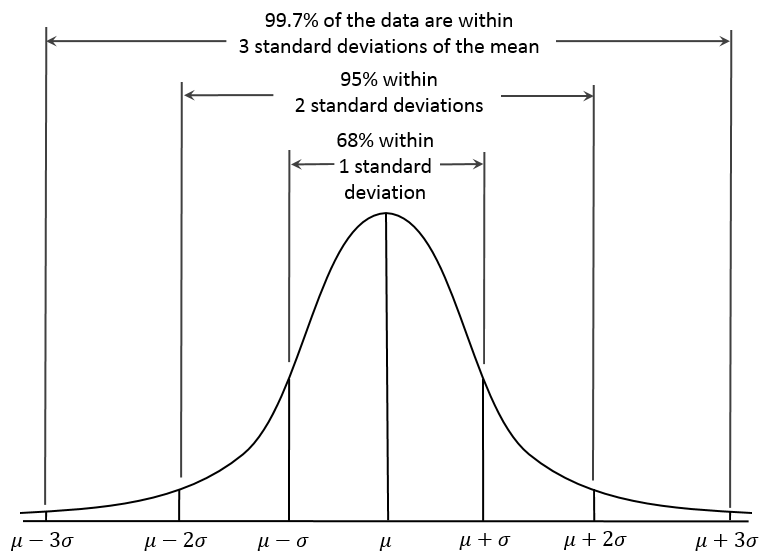
This rule tells us that around 68% of the data will fall within one standard deviation of the mean; around 95% will fall within two standard deviations of the mean; and 99.7% will fall within three standard deviations of the mean.
What percentile is 2 sigma
about 95 percent
One standard deviation, or one sigma, plotted above or below the average value on that normal distribution curve, would define a region that includes 68 percent of all the data points. Two sigmas above or below would include about 95 percent of the data, and three sigmas would include 99.7 percent.
How much data is within 2 standard deviations
Under this rule, 68% of the data falls within one standard deviation, 95% percent within two standard deviations, and 99.7% within three standard deviations from the mean.
What is the standard deviation rule
The empirical rule, or the 68-95-99.7 rule, tells you where your values lie: Around 68% of scores are within 1 standard deviation of the mean, Around 95% of scores are within 2 standard deviations of the mean, Around 99.7% of scores are within 3 standard deviations of the mean.
Is 1 standard deviation 68
The 68-95-99 rule
It says: 68% of the population is within 1 standard deviation of the mean. 95% of the population is within 2 standard deviation of the mean. 99.7% of the population is within 3 standard deviation of the mean.
Is one standard deviation always 34%
In normally distributed data, about 34% of the values lie between the mean and one standard deviation below the mean, and 34% between the mean and one standard deviation above the mean. In addition, 13.5% of the values lie between the first and second standard deviations above the mean.
What is the 67 95 99 rule
In statistics, the 68–95–99.7 rule, also known as the empirical rule, is a shorthand used to remember the percentage of values that lie within an interval estimate in a normal distribution: 68%, 95%, and 99.7% of the values lie within one, two, and three standard deviations of the mean, respectively.
What is the meaning of 1 sigma
In a normal distribution, it is postulated that things that are true 68% of the time are considered 1-Sigma events. Things that are true 95% of the time are considered 2-Sigma events and the three-Sigma rule implies that heuristically nearly all values lie within three standard deviations of the mean (3-Sigma).
What is the 68 96 99 rule
The 68-95-99 rule
It says: 68% of the population is within 1 standard deviation of the mean. 95% of the population is within 2 standard deviation of the mean. 99.7% of the population is within 3 standard deviation of the mean.
What is the 68-95-99 rule
In statistics, the 68–95–99.7 rule, also known as the empirical rule, is a shorthand used to remember the percentage of values that lie within an interval estimate in a normal distribution: 68%, 95%, and 99.7% of the values lie within one, two, and three standard deviations of the mean, respectively.
Is standard deviation always 68
The 68-95-99 rule
It says: 68% of the population is within 1 standard deviation of the mean. 95% of the population is within 2 standard deviation of the mean. 99.7% of the population is within 3 standard deviation of the mean.
Why is standard deviation always 1
We might have to do a little math to convert our data from one unit of measurement to another, but the thing we are measuring remains unchanged. When we convert our data into z scores, the mean will always end up being zero (it is, after all, zero steps away from itself) and the standard deviation will always be one.
What is the 68 95 99 rule
In statistics, the 68–95–99.7 rule, also known as the empirical rule, is a shorthand used to remember the percentage of values that lie within an interval estimate in a normal distribution: 68%, 95%, and 99.7% of the values lie within one, two, and three standard deviations of the mean, respectively.
What is the 69 97 99 rule
The 68-95-99 rule
It says: 68% of the population is within 1 standard deviation of the mean. 95% of the population is within 2 standard deviation of the mean. 99.7% of the population is within 3 standard deviation of the mean.
What is the 68 %- 95 rule
According to the 68 95 99 point 7 rule this means that there is an area of 95 percent contained within this interval. However. We are only interested in the area from 70 to 90.
Is 1-sigma good
For example, 1-sigma represents a poor level of quality, with 691,462 defects per million opportunities over time, which demonstrates a failure rate of over 69.1%. A level of 6-sigma represents a high level of quality, with 3.4 defects per million opportunities.
How much is 1-sigma
1 SIGMAcoin is 0.004546 US Dollar.
So, you've converted 1 SIGMAcoin to 0.004546 US Dollar. We used 219.987961 International Currency Exchange Rate. We added the most popular Currencies and CryptoCurrencies for our Calculator.
What is the 97 68 rule
The rule states that (approximately): – 68% of the data points will fall within one standard deviation of the mean. – 95% of the data points will fall within two standard deviations of the mean. – 99.7% of the data points will fall within three standard deviations of the mean. Created by Sal Khan.
What is the 68 88 99.7 rule
The 68-95-99 rule
It says: 68% of the population is within 1 standard deviation of the mean. 95% of the population is within 2 standard deviation of the mean. 99.7% of the population is within 3 standard deviation of the mean.
What is the 68 rule formula
Add and subtract the standard deviation to/from the mean: [m − s, m + s] is the interval that contains around 68% of data. Multiply the standard deviation by 2 : the interval [m − 2s, m + 2s] contains around 95% of data. Multiply the standard deviation by 3 . 99.7% of data falls in [m − 3s, m + 3s] .
What is the 68-95-99.7 rule multivariate
The empirical rule, or the 68-95-99.7 rule, tells you where most of the values lie in a normal distribution: Around 68% of values are within 1 standard deviation of the mean. Around 95% of values are within 2 standard deviations of the mean. Around 99.7% of values are within 3 standard deviations of the mean.
Is standard deviation always 1
The standard deviation of the z-scores is always 1. The graph of the z-score distribution always has the same shape as the original distribution of sample values. The sum of the squared z-scores is always equal to the number of z-score values.
Why is SD for z-score always 1
Z-scores have a SD (and a variance) of 1
That's because if a sum equals 1, the mean must equal 1 too.
What is the 65 98 99 rule
Sixty-eight percent of the data is within one standard deviation (σ) of the mean (μ), 95 percent of the data is within two standard deviations (σ) of the mean (μ), and 99.7 percent of the data is within three standard deviations (σ) of the mean (μ).
What is the 68.5 95 99 rule
The 68-95-99 rule
68% of the population is within 1 standard deviation of the mean. 95% of the population is within 2 standard deviation of the mean. 99.7% of the population is within 3 standard deviation of the mean.