What is log base 10 called
In mathematics, the common logarithm is the logarithm with base 10. It is also known as the decadic logarithm and as the decimal logarithm, named after its base, or Briggsian logarithm, after Henry Briggs, an English mathematician who pioneered its use, as well as standard logarithm.
What is the basic formula for logarithms
logarithm, the exponent or power to which a base must be raised to yield a given number. Expressed mathematically, x is the logarithm of n to the base b if bx = n, in which case one writes x = logb n. For example, 23 = 8; therefore, 3 is the logarithm of 8 to base 2, or 3 = log2 8.
What is log in math
log: (in math) An abbreviation for logarithm. logarithm: The power (or exponent) to which one base number must be raised — multiplied by itself — to produce another number. For instance, in the base 10 system, 10 must be multiplied by 10 to produce 100. So the logarithm of 100, in a base 10 system, is 2.
What is a base e logarithm called
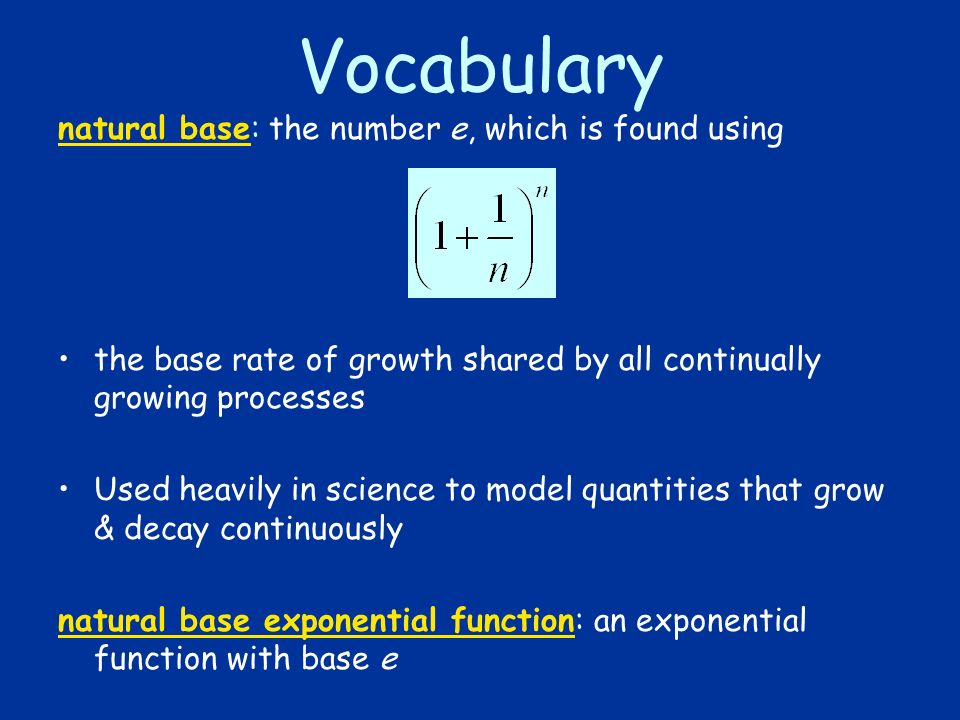
The logarithms having base e are termed as natural logarithms. loge x is a natural logarithm because the base is e. Natural logarithms are generally written as ln x. The Latin name of natural logarithms is logarithmus naturali, giving the abbreviation ln.
Does log mean base 10 or base e
The difference between log and ln is that log is defined for base 10 and ln is denoted for base e.
Why are logarithms important
Logarithmic functions are important largely because of their relationship to exponential functions. Logarithms can be used to solve exponential equations and to explore the properties of exponential functions.
How are logarithms used in real life
Much of the power of logarithms is their usefulness in solving exponential equations. Some examples of this include sound (decibel measures), earthquakes (Richter scale), the brightness of stars, and chemistry (pH balance, a measure of acidity and alkalinity).
Why are logarithms useful
Logarithms can be used to solve exponential equations and to explore the properties of exponential functions. They will also become extremely valuable in calculus, where they will be used to calculate the slope of certain functions and the area bounded by certain curves.
What do you call the logarithm with a base e
The logarithms having base e are termed as natural logarithms.
What is e used for in real life
It is commonly used in a wide range of applications, including population growth of living organisms and the radioactive decay of heavy elements like uranium by nuclear scientists. It can also be used in trigonometry, probability, and other areas of applied mathematics.
What is the difference between base 10 and base e
While the base of a common logarithm is 10, the base of a natural logarithm is the special number e. Although this looks like a variable, it represents a fixed irrational number approximately equal to 2.718281828459. (Like pi, it continues without a repeating pattern in its digits.)
How logarithm helped in making our life easier
Logarithms in Real Life
Logarithms simplify insights involving large figures, such as the number of visits per day on Google's search home page, earthquake intensity readings, or sound intensity readings of a commercial airplane during take off.
How did logarithms change the world
Mathematical tables containing common logarithms (base-10) were extensively used in computations prior to the advent of computers and calculators, not only because logarithms convert problems of multiplication and division into much easier addition and subtraction problems, but for an additional property that is unique …
Why are logarithms so useful
Logarithmic functions are important largely because of their relationship to exponential functions. Logarithms can be used to solve exponential equations and to explore the properties of exponential functions.
How important is logarithm in real life problems
Much of the power of logarithms is their usefulness in solving exponential equations. Some examples of this include sound (decibel measures), earthquakes (Richter scale), the brightness of stars, and chemistry (pH balance, a measure of acidity and alkalinity).
How do you use base e
"e" is a commonly used base for exponential functions. Any number greater than 0 (except for 1) can be used for an exponential function. "e", called "Euler's Constant" (pronounced oiler) is one of the most commonly used bases, i.e. y=ex .
Why is natural log important
The natural log is the logarithm to the base of the number e and is the inverse function of an exponential function. Natural logarithms are special types of logarithms and are used in solving time and growth problems. Logarithmic functions and exponential functions are the foundations of logarithms and natural logs.
Why is e so important
It is often called Euler's number after Leonhard Euler (pronounced "Oiler"). e is an irrational number (it cannot be written as a simple fraction). e is the base of the Natural Logarithms (invented by John Napier). e is found in many interesting areas, so is worth learning about.
Where is base e used
The natural base e is defined as an irrational number whose approximate value is 2.7182818284. It is commonly used as the base of logarithmic and exponential functions and in compounding interest situations.
Why are logarithms important in real life
Much of the power of logarithms is their usefulness in solving exponential equations. Some examples of this include sound (decibel measures), earthquakes (Richter scale), the brightness of stars, and chemistry (pH balance, a measure of acidity and alkalinity).
What is the purpose of logarithms in real life
Logarithms are used for measuring the magnitude of earthquakes. Logarithms are used for measuring the noise levels in dBs (decibels). They are used to measure the pH level of chemicals. Logarithms are used in radioactivity, mainly to detect the half life of a radioactive element.
Why are logarithms useful in real life
Much of the power of logarithms is their usefulness in solving exponential equations. Some examples of this include sound (decibel measures), earthquakes (Richter scale), the brightness of stars, and chemistry (pH balance, a measure of acidity and alkalinity).
How do logarithms help in real life
Logarithms are used for measuring the magnitude of earthquakes. Logarithms are used for measuring the noise levels in dBs (decibels). They are used to measure the pH level of chemicals. Logarithms are used in radioactivity, mainly to detect the half life of a radioactive element.
What are 2 real life examples that logarithm is useful
Much of the power of logarithms is their usefulness in solving exponential equations. Some examples of this include sound (decibel measures), earthquakes (Richter scale), the brightness of stars, and chemistry (pH balance, a measure of acidity and alkalinity).
What does base e logarithm mean
The natural logarithm of a number is its logarithm to the base of the mathematical constant e, which is an irrational and transcendental number approximately equal to 2.718281828459. The natural logarithm of x is generally written as ln x, loge x, or sometimes, if the base e is implicit, simply log x.